CONSTRUCTING QUANTUM ANGULAR MOMENTUM L3 IN SPECIFIC DIRECTION BY USING U(1) GROUP

Article Sidebar

PDF
Published: Sep 30, 2024
DOI: https://doi.org/10.22452/mjs.vol43no3.7
Keywords:
group character group group representation quantum angular momentum

Main Article Content

Ahmad Aqwa Rosli
Laboratory of Computational Sciences and Mathematical Physics, Institute for Mathematical Research (INSPEM), Universiti Putra Malaysia, 43400 Serdang, Selangor, MALAYSIA.
https://orcid.org/0009-0007-0486-522X
Nurisya Mohd Shah
Department of Physics, Faculty of Scinec, Universiti Putra Malaysia, 43400 Serdang, Selangor, MALAYSIA.
https://orcid.org/0000-0001-7783-1118

Abstract

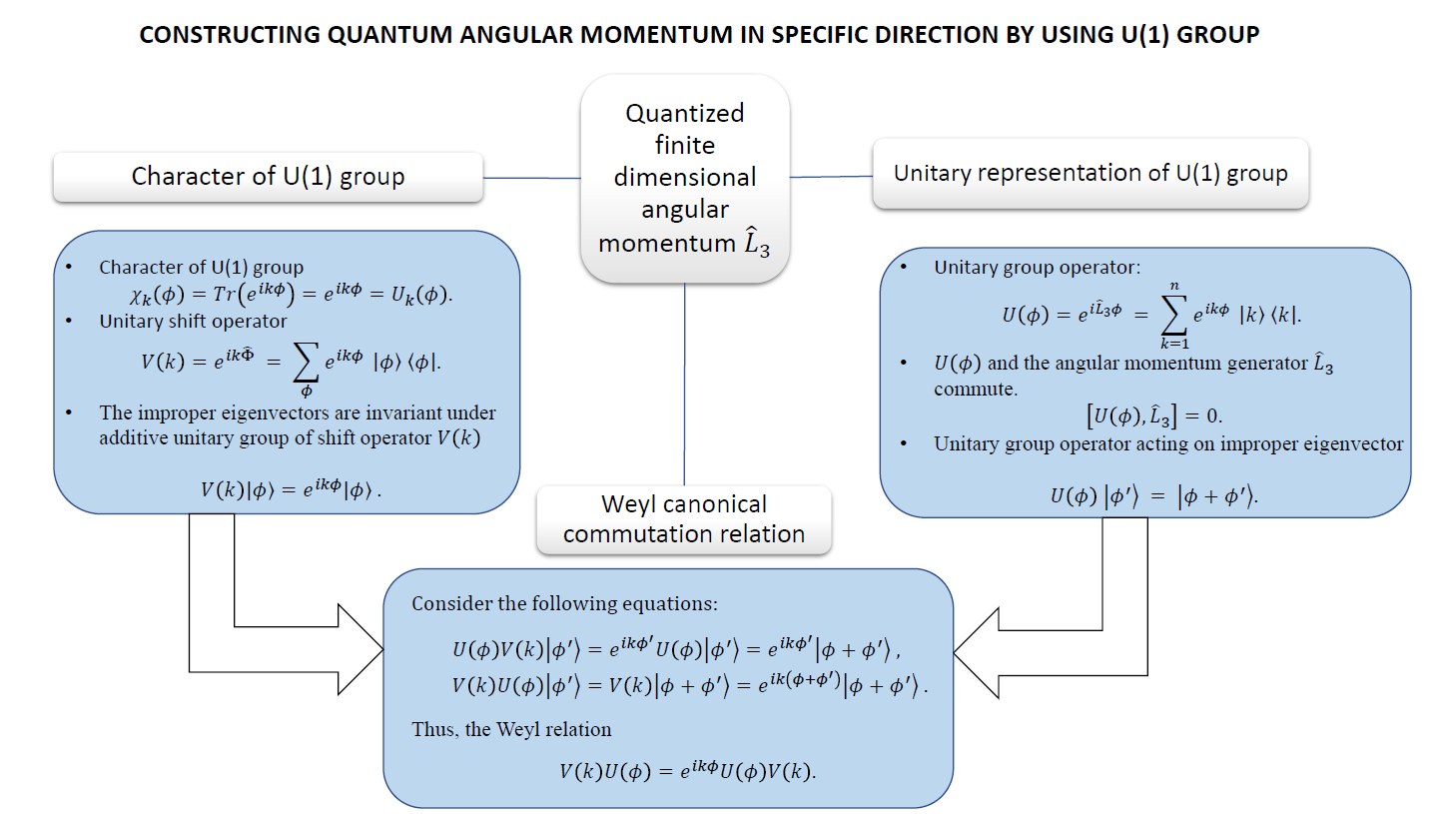
The purpose of this work is to investigate the mathematical structure of finite quantum angular momentum in a specific direction L3 which can be constructed from the representation of the U(1)  group. The angular momentum eigenstate is invariant under Abelian rotation symmetry. The character group of  U(1) is constructed to show that there exists an additive unitary operator for the angular momentum eigenstate, and the rotation eigenstate is invariant under it. The Weyl relation is proved by showing that the angle and angular momentum L3 are the canonical conjugate pair of observables.

Downloads

Download data is not yet available.

Article Details

How to Cite
Rosli, A. A., & Mohd Shah, N. (2024). CONSTRUCTING QUANTUM ANGULAR MOMENTUM L3 IN SPECIFIC DIRECTION BY USING U(1) GROUP. Malaysian Journal of Science, 43(3), 56–58. https://doi.org/10.22452/mjs.vol43no3.7
Issue
Vol 43 No 3 (2024): September 2024
Section
Original Articles

Licensee MJS, Universiti Malaya, Malaysia. This article is an open-access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).

References

Berry, M. V. (1977). Semi-classical mechanics in phase space: a study of Wigner’s function. Philosophical Transactions of the Royal Society of London. Series A, Mathematical and Physical Sciences, 287(1343), 237-271.

Bizarro J. P. (1994). Weyl-Wigner formalism for rotation-angle and angular-momentum variables in quantum mechanics, Physical Review A, 49(5):3255.

Busch P., Grabowski M., Lahti P. J. (1997). Operational quantum physics (Vol. 31). Springer Science & Business Media.

Das A. (2020). Lectures on quantum field theory. World Scientific.

Dirac P. A. M. (1981). The principles of quantum mechanics (No. 27). Oxford University Press.

Hall B. C. (2013). Quantum theory for mathematicians. Springer.

Isham C. J. (1984). Topological and global aspects of quantum theory. In Relativity, groups and topology. 2. edited by B. S. Dewitt and R. Stora, North-Holland: Amsterdam, 1984, pp. 1059- 1290.

Iwai T. (1982). The symmetry group of the harmonic oscillator and its reduction. Journal of Mathematical Physics, 23(6):1088-1092.

Sundermeyer K. (1982). Constrained dynamics with applications to Yang-Mills theory, general relativity, classical spin, dual string model. New York, USA: Springer-Verlag.

Weinbub, J., & Ferry, D. K. (2018). Recent advances in Wigner function approaches. Applied Physics Reviews, 5(4).

Woit, P., Woit, & Bartolini. (2017). Quantum theory, groups, and representations. New York, NY, USA: Springer International Publishing.

Yamada K. (1982). Angular-momentum—angle commutation relations and minimum-uncertainty states. Physical Review D, 25(12):3256.

Zhang, K. Y., & Feng, W. Z. (2023). Explaining the W boson mass anomaly and dark matter with a U (1) dark sector. Chinese Physics C, 47(2), 023107.